how to tell if box plot is normally distributed IF the box plot is relatively short, then the data is more compact. If the box plot is relatively tall, then the data is spread out. The interpretation of the compactness or spread of . Sigma's weatherproof round extension ring increases the capacity of a weatherproof box. The rugged, die-cast construction prevents moisture penetration making the boxes suitable for wet, .
0 · skewed to the right boxplot
1 · positively skewed distribution box plot
2 · positively skewed box plots
3 · positive skew vs negative boxplot
4 · how to interpret boxplot results
5 · boxplot skewed to the left
6 · box and whiskers chart explained
7 · 25th percentile on a boxplot
Shop for metal palettes and watercolor boxes for holding half pans,full pans and tubes. Handy for travelling watercolorists. Lowest online prices guaranteed.
skewed to the right boxplot
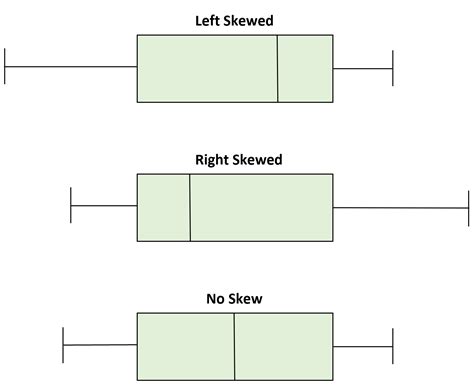
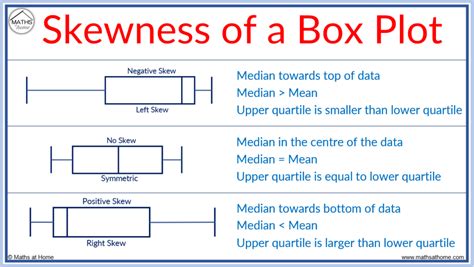
To determine whether a distribution is skewed in a box plot, look at where the median line falls within the box and whiskers. You have a symmetrical distribution when the box centers approximately on the median line, and the upper and .Normal Distribution : If a box plot has equal proportions around the median, we can say distribution is symmetric or normal. Positively Skewed : For a distribution that is positively skewed, the box plot will show the median closer to the lower . IF the box plot is relatively short, then the data is more compact. If the box plot is relatively tall, then the data is spread out. The interpretation of the compactness or spread of . For quick and visual identification of a normal distribution, use a QQ plot if you have only one variable to look at and a Box Plot if you have many. Use a histogram if you need to present your results to a non-statistical public.
A box plot (aka box and whisker plot) uses boxes and lines to depict the distributions of one or more groups of numeric data. Box limits indicate the range of the central 50% of the data, with .A box plot is a diagram used to display the distribution of data. A box plot indicates the position of the minimum, maximum and median values along with the position of the lower and upper quartiles. From this, the range, interquartile .A distribution may not look normally distributed from the histogram, but it still may be normally distributed. Outliers : For a normal distribution, there should not be more than one outlier. One .
A boxplot is a standardized way of displaying the distribution of data based on its five-number summary (“minimum”, first quartile [Q1], median, third quartile [Q3] and “maximum”). Boxplots can tell you about your outliers and their values, if .One way to understand a box plot is to think of what a box plot of data from a normal distribution will look like. The graph below shows a standard normal probability density function ruled into four quartiles, and the box plot you would .Normal Distribution : If a box plot has equal proportions around the median, we can say distribution is symmetric or normal. Positively Skewed : For a distribution that is positively skewed, the box plot will show the median closer to the lower . You only have 101 data points. In such a situation, it often makes sense to plot the raw data instead of summary graphics like histograms or smoothed density plots. The raw data can be shown using q-q-plots, as you .
Note the language. The shorthand (used above) is to test the assumption that the residuals are normally distributed. What this really means is testing the assumption that the residuals are sampled from a normal distribution, or are . How to Draw a Normal Probability Plot By Hand. Arrange your x-values in ascending order (smallest to largest). Calculate f i = (i – 0.375)/(n + 0.25), where i is the position of the data value in the ordered list and n is the number of observations.; Find the z-score for each f i; Plot . Using a box plot. A box plot for a normal distribution shows that the mean is the same as the median. It also shows that the data has no extreme values. The data will be symmetrical. Take a look at the two box plots in Figures 8 and 9 below. The data in Figure 8 is from a nearly normal distribution. The data in Figure 9 is from a non-normal .
How to tell if data set is normally distributed? Hi I’m writing a research paper to assess the effectiveness of a medical conference. We used a pre and post conference questionnaire which the delegates answered on the contents of the conference so we can look at how effective the conference was at educating them. I'm trying to plot box plots with normal distribution of the underlying data next to the plots in a vertical format like this: This is what I currently have graphed from an excel sheet uploaded to R: And the code associated with them:
Gauss–Markov theorem. In statistics, the Gauss–Markov theorem (or simply Gauss theorem for some authors) states that the ordinary least squares (OLS) estimator has the lowest sampling variance within the class of linear unbiased estimators, if the errors in the linear regression model are uncorrelated, have equal variances and expectation value of zero.
And so a normal distribution might be quite appropriate for describing Spring temperatures. [1] Fig. 48 Illustration of what happens when you change the mean of a normal distribution. The solid line depicts a normal distribution with a mean of μ = 4. The dashed line shows a normal distribution with a mean of μ = 7.
A commonly used graphical procedure is to make a 'normal probability plot', also called a 'Q-Q plot' (or 'quantile-quantile' plot). Roughly speaking, the points on a normal probability plot should lie in a straight line (with some recognition that it is not unusual for a few of the smaller or larger observations to stray from the line).
The following plot shows a boxplot of data with a normal distribution and a box plot of data with a log normal distribution. The plots show that the distribution between the data points is different. The first and second quartiles are very short compared to the first and second quartiles of the normal distribution example, and compared to the . I am trying to know if the below box-plot represents a normal distribution or if its similar to a normal but i have some doubts about it. The median is 2.0, mean is 2.5 and sd is 1.60. Although the box is symmetric the lengths of whisker are not also mean is not equal to median so i would say this is not similar to a gaussian distribution but i .Under Plots, we can choose a histogram and/or density plot (figure on the left) or boxplot and/or violin plot and/or data points (figure on the right). We can just look at this data and visually inspect with our eyes whether the data is normally distributed based on the density curve.B. Know how to calculate percentiles from a normal distribution. C. Understand how normal n-quantiles are used to divide the area under a normal curve into n-equal areas. D. Understand the basic construction of a normal quantile plot. E. Use a normal quantile plot to assess whether data are from a normal distribution.
A boxplot, also known as a box plot, box plots, or box-and-whisker plot, is a standardized way of displaying the distribution of a data set based on its five-number summary of data points: the “minimum,” first quartile [Q1], .
positively skewed distribution box plot
Step #4: Compute the normal distribution values for every x-axis value. Now, find the normal distribution values—the probability of a student getting a certain exam score represented by a particular x-axis value—for . A box plot is a type of plot that displays the five number summary of a dataset, which includes:. The minimum value; The first quartile (the 25th percentile) The median value; The third quartile (the 75th percentile) The maximum value; To make a box plot, we first draw a box from the first to the third quartile.
We can see that this dataset doesn’t follow the common bell-shaped distribution, indicating that the data don’t follow a normal distribution. Using a Q-Q Plot to Check if a Distribution is Normally Distributed. A common plot used to check if data are normally distributed is a Quantile-Quantile plot (or Q-Q plot, for short). Let’s look at the histogram and the box plot for Variable 1. The distribution appears to be approximately normal. The histogram is mounded in shape, and the tails are symmetric. In the box plot, the mean and the median are close to one another, the median is close to the center of the box, and the whiskers are about the same length.My data is based on a number of Kaplan Meier plots. Now I know that Kaplan Meier plots are based on data where an event either happens or doesn't happen at a specific time. As far as I understand that would be binomialy distributed as the event either happens or doesn't.Boxplots for normally distributed data (top) and non-normal data (bottom). 2. Boxplot. Draw a boxplot of your data. If your data comes from a normal distribution, the box will be symmetrical with the mean and median in the center. If the data meets the assumption of normality, there should also be few outliers.
So, to the point u/malluss made, it’s more likely that you’re applying a rule that identifies an outlier based on outlier criteria for a standard normal distribution, when in fact your data does not follow that distribution as currently measured. A transformation will help you adjust the view of your measured distribution to fit the rules . Also, if you have extremely large sample sizes then statistical tests like the Shapiro-Wilk test will almost always tell you that your data is non-normal. For this reason, it’s often best to inspect your data visually using graphs like histograms and Q-Q plots. . #Create box plots that show distribution of weight loss for each group boxplot . Using a box plot. A box plot for a normal distribution shows that the mean is the same as the median. It also shows that the data has no extreme values. The data will be symmetrical. Take a look at the two box plots in Figures 8 and 9 below. The data in Figure 8 is from a nearly normal distribution. The data in Figure 9 is from a non-normal .
I am not sure whether the points are Scatter plot. And it is easy to draw points. So I just plot the box chart with norm distribution curve. It is mainly based on Rotated Normal Distribution and some boxplot settings can be found in chapter 5.12.1 of Manual. $\begingroup$ Note that the normality test will not tell you "this data is normally distributed" - you can only fail to reject a null hypothesis, not confirm it. The test can only tell you "there is insufficient evidence to conclude this data is not normally distributed". That can happen either because 1) the data is indeed normally distributed, or 2) the data is not normally .For example, the normal probability Q-Q plot below displays a dataset with 5000 observations along with the normality test results. The p-value for the test is 0.010, which indicates that the data do not follow the normal distribution. However, the points on the graph clearly follow the distribution fit line.

mk4 vr6 12v gruvenparts metal oil filter housing
$31.45
how to tell if box plot is normally distributed|how to interpret boxplot results