box muller joint distribution • Weisstein, Eric W. "Box-Muller Transformation". MathWorld.• How to Convert a Uniform Distribution to a Gaussian Distribution (C Code) See more It allows the user to calculate the weights of carbon steel, stainless steel, copper alloys and aluminium alloy products of various forms such as flat sections, shapes and hollow sections such as tube and pipe.
0 · proof of box muller
1 · how to calculate box muller
2 · box-muller method exercise
3 · box muller wikipedia
4 · box muller method pdf
5 · box muller method formula
6 · box muller maths
7 · box muller formula
Robert Weiss and Donald Johnson began Weiss-Johnson Sheet Metal Ltd. in 1977 with nothing more than a pick-up truck, a box of business cards and a bank loan.
The Box–Muller transform, by George Edward Pelham Box and Mervin Edgar Muller, is a random number sampling method for generating pairs of independent, standard, normally distributed (zero expectation, unit variance) random numbers, given a source of uniformly distributed random numbers. The . See moreSuppose U1 and U2 are independent samples chosen from the uniform distribution on the unit interval (0, 1). Let See moreThe polar method differs from the basic method in that it is a type of rejection sampling. It discards some generated random numbers, but can be faster than the basic method . See more• Inverse transform sampling• Marsaglia polar method, similar transform to Box–Muller, which uses Cartesian coordinates, instead of polar coordinates See more
• Weisstein, Eric W. "Box-Muller Transformation". MathWorld.• How to Convert a Uniform Distribution to a Gaussian Distribution (C Code) See moreThe polar form was first proposed by J. Bell and then modified by R. Knop. While several different versions of the polar method have been described, the version of R. Knop will be . See more
C++The standard Box–Muller transform generates values from the standard normal distribution (i.e. standard normal deviates) with mean 0 and standard deviation 1. The implementation below in standard See more Exercise (Box–Muller method): Let U and V be independent random variables that are uniformly distributed on [0, 1]. Define X: = √− 2log(U)cos(2πV) and Y: = √− .
cnc machine installation cost
1 Box Muller It would be nice to get a standard normal from a standard uniform by inverting the distribution function, but there is no closed form formula for this distribution function N(x) = P(X . A transformation which transforms from a two-dimensional continuous uniform distribution to a two-dimensional bivariate normal distribution (or complex normal distribution).Transformation Methods: Box-Muller Algorithm I Proposition. If R2 ˘Exp(1 2) and ˘U[0;2ˇ] are independent then X= Rcos , Y = Rsin are independent with X˘N(0;1); Y ˘N(0;1): Proof: We . The Box–Muller transform is a pseudo-random number sampling method for generating pairs of independent, standard, normally distributed (zero expectation, unit variance) random numbers, given a source of uniformly .
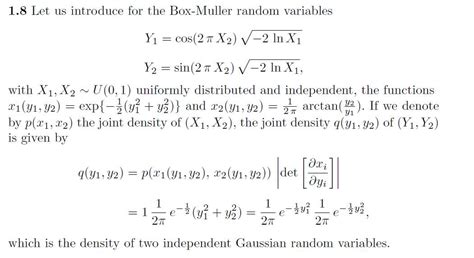
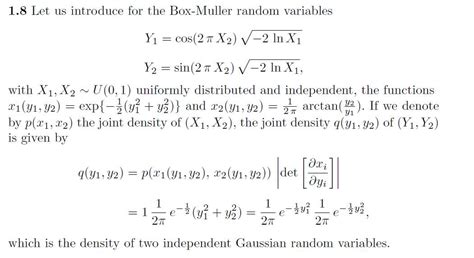
One can show that the density of R is fR(r) = re − r2 / 2 and the density of Θ is fΘ(θ) = θ / 2π. So the joint density of R and Θ is fR, Θ(r, θ) = re − r2 / 2 / 2π. Box-Muller transform is a method used to produce a normal distribution. Imagine two independent distributions of X, Y ~N (0,1) plotted in the Cartesian field. Then, we need some distance. Here we want to show that the Box-Muller method generates a pair of independent standard Gaussian random variables. But I don't understand why we use the determinant? For me when you have two indep. Recalling now that the joint pdf of two independent uniforms is the unit square, we have that the joint distribution of the transformatons is. f(z0, z1) = 1 2πexp{− 1 2(z20 + z21)}, − .
The Box–Muller transform, by George Edward Pelham Box and Mervin Edgar Muller, [1] is a random number sampling method for generating pairs of independent, standard, normally distributed (zero expectation, unit variance) random numbers, given a source of uniformly distributed random numbers.
Exercise (Box–Muller method): Let U and V be independent random variables that are uniformly distributed on [0, 1]. Define X: = √− 2log(U)cos(2πV) and Y: = √− 2log(U)sin(2πV). Show that X and Y are independent and N0, 1 -distributed.
1 Box Muller It would be nice to get a standard normal from a standard uniform by inverting the distribution function, but there is no closed form formula for this distribution function N(x) = P(X < x) = √1 2π R x −∞ e−x02/2dx0. The Box Muller method is a brilliant trick to overcome this by producing two independent standard normals A transformation which transforms from a two-dimensional continuous uniform distribution to a two-dimensional bivariate normal distribution (or complex normal distribution).Transformation Methods: Box-Muller Algorithm I Proposition. If R2 ˘Exp(1 2) and ˘U[0;2ˇ] are independent then X= Rcos , Y = Rsin are independent with X˘N(0;1); Y ˘N(0;1): Proof: We have f R2; (r 2 ) = 1 2 exp r2=2 1 2ˇ and therefore we are interested in f X;Y(x;y) = f R2; (r 2(x;y); (x;y)) 2 det @(r; ) @(x;y) where 2 det @(r; ) @(x;y) @r . The Box–Muller transform is a pseudo-random number sampling method for generating pairs of independent, standard, normally distributed (zero expectation, unit variance) random numbers, given a source of uniformly distributed random numbers.
One can show that the density of R is fR(r) = re − r2 / 2 and the density of Θ is fΘ(θ) = θ / 2π. So the joint density of R and Θ is fR, Θ(r, θ) = re − r2 / 2 / 2π.
Box-Muller transform is a method used to produce a normal distribution. Imagine two independent distributions of X, Y ~N (0,1) plotted in the Cartesian field. Then, we need some distance. Here we want to show that the Box-Muller method generates a pair of independent standard Gaussian random variables. But I don't understand why we use the determinant? For me when you have two indep. Recalling now that the joint pdf of two independent uniforms is the unit square, we have that the joint distribution of the transformatons is. f(z0, z1) = 1 2πexp{− 1 2(z20 + z21)}, − ∞
The Box–Muller transform, by George Edward Pelham Box and Mervin Edgar Muller, [1] is a random number sampling method for generating pairs of independent, standard, normally distributed (zero expectation, unit variance) random numbers, given a source of uniformly distributed random numbers.
Exercise (Box–Muller method): Let U and V be independent random variables that are uniformly distributed on [0, 1]. Define X: = √− 2log(U)cos(2πV) and Y: = √− 2log(U)sin(2πV). Show that X and Y are independent and N0, 1 -distributed.1 Box Muller It would be nice to get a standard normal from a standard uniform by inverting the distribution function, but there is no closed form formula for this distribution function N(x) = P(X < x) = √1 2π R x −∞ e−x02/2dx0. The Box Muller method is a brilliant trick to overcome this by producing two independent standard normals
A transformation which transforms from a two-dimensional continuous uniform distribution to a two-dimensional bivariate normal distribution (or complex normal distribution).Transformation Methods: Box-Muller Algorithm I Proposition. If R2 ˘Exp(1 2) and ˘U[0;2ˇ] are independent then X= Rcos , Y = Rsin are independent with X˘N(0;1); Y ˘N(0;1): Proof: We have f R2; (r 2 ) = 1 2 exp r2=2 1 2ˇ and therefore we are interested in f X;Y(x;y) = f R2; (r 2(x;y); (x;y)) 2 det @(r; ) @(x;y) where 2 det @(r; ) @(x;y) @r . The Box–Muller transform is a pseudo-random number sampling method for generating pairs of independent, standard, normally distributed (zero expectation, unit variance) random numbers, given a source of uniformly distributed random numbers.
One can show that the density of R is fR(r) = re − r2 / 2 and the density of Θ is fΘ(θ) = θ / 2π. So the joint density of R and Θ is fR, Θ(r, θ) = re − r2 / 2 / 2π.
Box-Muller transform is a method used to produce a normal distribution. Imagine two independent distributions of X, Y ~N (0,1) plotted in the Cartesian field. Then, we need some distance.
Here we want to show that the Box-Muller method generates a pair of independent standard Gaussian random variables. But I don't understand why we use the determinant? For me when you have two indep.
proof of box muller
Klippon® POK enclosures are made from high-quality glass-fibre reinforced polyester. They are suited for applications and environments where corrosion and impact resistance, as well as a high IP protection, are required. The range is .
box muller joint distribution|box muller maths